The Butterfly Effect
 The
"Butterfly Effect" is the propensity
of a system to be sensitive to initial conditions.Such systems over
time become unpredictable,this idea gave rise to the notion of a butterfly
flapping it's wings in one area of the world,causing a tornado or some such
weather event to occur in another remote area of the world.
The
"Butterfly Effect" is the propensity
of a system to be sensitive to initial conditions.Such systems over
time become unpredictable,this idea gave rise to the notion of a butterfly
flapping it's wings in one area of the world,causing a tornado or some such
weather event to occur in another remote area of the world.
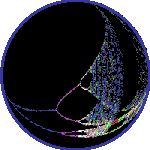
Comparing this effect to the domino effect,is slightly
misleading.There is dependence on the initial sensitivity,but whereas a simple
linear row of dominoes would cause one event to initiate another similar
one,the butterfly effect amplifies the condition upon
each iteration.
The butterfly effect has been most commonly associated with the Weather
system as this is where the
 discovery of
"non-linear"
phenomenon began when
Edward Lorenz found anomalies in computer
models of the weather.But
Henri Poincaré had already
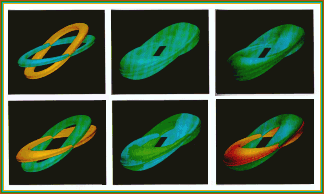
made inroads into this area. Mapping the results in
"phase space" produced a two-lobe map called the
Lorenz Attractor. The word
attractor meaning that events tended
to be attracted towards the two lobes,and events outside of the lobes are
such things like snow in the desert.
discovery of
"non-linear"
phenomenon began when
Edward Lorenz found anomalies in computer
models of the weather.But
Henri Poincaré had already
made inroads into this area. Mapping the results in
"phase space" produced a two-lobe map called the
Lorenz Attractor. The word
attractor meaning that events tended
to be attracted towards the two lobes,and events outside of the lobes are
such things like snow in the desert.
The attractor acts like an egg whisk,teasing apart parameters that
may initially be close together,this is why the weather is so hard to predict.
 Super computers run several models of the weather in
parallel to discover whether they stay close together or diverge away from
each other.Models that stay similar in nature give an indication that the
weather is relatively predictable,and are used to indicate the confidence
level that Meteorologists have in a prediction.
Super computers run several models of the weather in
parallel to discover whether they stay close together or diverge away from
each other.Models that stay similar in nature give an indication that the
weather is relatively predictable,and are used to indicate the confidence
level that Meteorologists have in a prediction.
It is not just the weather though that is subject to such
phenomena.Any
"Newtonian Classical" system where one system
is in competition with another,such as the
"Chaotic Pendulum" which plays magnetism
off against gravity will exhibit "sensitivity to initial
conditions".
 Animal populations may also be subject to the same
phenomena.Work done by Robert May,suggests that
predator-prey systems have complex dynamics making
them prone to "boom" and "bust",due to the difference equations that model
them.Such a system even with two variables such as
Rabbits and Foxes
can create a system that is much more complex than would be thought to
be the case.Lack of Foxes means that the Rabbit population can increase,but
increasing numbers of Rabbits means Foxes have more food and are likely to
survive and reproduce,which in turn decreases the number of Rabbits.It is
possible for such systems to find a steady state or equilibrium,and even
though species can become extinct,there is a tendency for
populations to be robust,but they
can vary dramatically under certain circumstances. Real populations of
course,have more than two variables making them ever more complex.But as
can be seen from the diagram, such systems are not as simple as might be
thought.
Animal populations may also be subject to the same
phenomena.Work done by Robert May,suggests that
predator-prey systems have complex dynamics making
them prone to "boom" and "bust",due to the difference equations that model
them.Such a system even with two variables such as
Rabbits and Foxes
can create a system that is much more complex than would be thought to
be the case.Lack of Foxes means that the Rabbit population can increase,but
increasing numbers of Rabbits means Foxes have more food and are likely to
survive and reproduce,which in turn decreases the number of Rabbits.It is
possible for such systems to find a steady state or equilibrium,and even
though species can become extinct,there is a tendency for
populations to be robust,but they
can vary dramatically under certain circumstances. Real populations of
course,have more than two variables making them ever more complex.But as
can be seen from the diagram, such systems are not as simple as might be
thought.
The chemical world is also not free from such intrusions of
 non-linearity.In certain cases chemical
feedback produces effects as that in the
Belousov-Zhabotinsky reaction, creating
concentric rings, which are produced by a chemical change, whose decision
to change from one state to another cannot be predicted.The
B-Z chemical system is currently being
trialled as a means to achieve artificially intelligent states in
robots.
non-linearity.In certain cases chemical
feedback produces effects as that in the
Belousov-Zhabotinsky reaction, creating
concentric rings, which are produced by a chemical change, whose decision
to change from one state to another cannot be predicted.The
B-Z chemical system is currently being
trialled as a means to achieve artificially intelligent states in
robots.
Phase space portraits of liquid flow show that they too are subject to the
same kind of non-linearity that is inherent in other physical systems.It
may be apparent when turning on a tap that sporadic drips become "laminar"
as the flow increases.What might not be apparent is the nature of the change
from semi-random to continuous.It may seem rather at odds with intuition
that such natural systems have inherent behaviour that is not random,or indeed
that is not capable of being predicted.It may also seem that "not random"
means "predictable".
Natural systems can present a tangled mix of determinism and
randomness,or "order" and
 "chaos".In
such cases as water moving from drips to continuous flow, pictures called
"Bifurcation diagrams" demonstrate the nature of movement from order into
chaos.This bifurcation is based on Robert May's work,but one of the intriguing
things about bifurcations is that the same pattern occurs no matter what
system is iterated.In fact
Mitchell
"chaos".In
such cases as water moving from drips to continuous flow, pictures called
"Bifurcation diagrams" demonstrate the nature of movement from order into
chaos.This bifurcation is based on Robert May's work,but one of the intriguing
things about bifurcations is that the same pattern occurs no matter what
system is iterated.In fact
Mitchell
 Feigenbaum discovered that there was a "constant of doubling"
hidden in amongst all these systems.
Feigenbaum discovered that there was a "constant of doubling"
hidden in amongst all these systems.
Electronic apparatus is also not free from such effects,and it is perhaps
ironic,that we think of electronic apparatus as as being the epitome
of predictable determinism and ruthless clockwork efficiency.Indeed the powerful
computers used to predict weather,would seem ineffectual if they were not
ruthless automatons.But such effects occur only in certain circumstances
where there is "sensitivity to initial conditions".Amplifiers for
instance,produce a howl when feedback occurs as they go into a stable
state of oscillation.Logic gates as used in computers
have to select a "0" or a "1",and this relies on choosing between two
states whose boundary is indeterminate,and it is when a computer confuses
a "0" for a "1" or vice versa that mistakes occur.
 |
 |
Phase space portrait of regular
flow in a Taylor-Couette system |
Phase space portrait of
chaotic flow as the attractor
becomes "strange" |
Many of the shapes that describe non-linear systems are fractal,a set of
shapes that are self-similar on smaller and smaller scales with no limit
to the size of the scale. Fractals were discovered by
Benoit Mandelbrot at IBM.
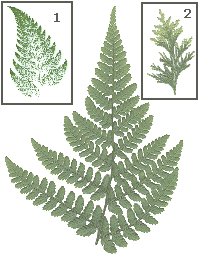 |
| A picture of a real fern and an affine transformation
performed by "Winfract" (Inset1).Inset 2 is a real image of a conifer,and
bears an uncanny resemblance to a diffusion limited
aggregate. |
Fractals have been seen as describing naturally occurring phenomena
such as the cragginess of mountains or the shapes of certain plant forms,
such as ferns,which can be modelled by affine transformations.
Whether in fact Nature is fractal,or whether it just describes it better
than the simple geometry of Euclid depends on the
philosophical view taken of mathematics as a whole. Some people think
mathematics is just a tool or a creation of man,and
therefore Nature is only described or mapped by mathematics.
Others think that the description is real -at least in the sense that the
similarity is not superficial,that in fact natural objects that look fractal,or
which fractals look like,are similar in appearance because
at some fundamental level the natural objects are
obeying some form of rule system that bears a similarity to the
sort of rules which govern fractals.
Whichever way you look at it,one thing no one can say is that mathematics
is irrelevant to Nature. From butterflies to plants,from the weather
to chemistry,mathematics is modelling or displaying attributes of Nature,and
helping us to understand what we see.
A new conception was being made....that
whatever fundamental units the world is put together from,they are more
delicate,more fugitive,more startling than we catch in the Butterfly Net
of our senses.
Jacob Bronowski "The Ascent of Man"
How to Float Like a Butterfly
The intricacies of insect flight are astounding. But the animals' small size
and swift movements make detailed studies of their aerodynamic acrobatics
difficult. Now results of a study of free-flying butterflies published today
in the journal Nature suggest that the insects rely on a variety of techniques,
often employed in successive strokes, over the course of a flight. R. B.
Srygley and Adrian L.R. Thomas of the University of Oxford trained red admiral
butterflies to fly toward a fake flower at the end of a wind tunnel. Photographs
snapped as the insects moved through wisps of smoke in the chamber provided
the researchers with an opportunity to analyze their flight patterns.
Specifically, the scientists matched patterns in the airflow around the
butterflies' wings to standard mathematical patterns with known properties.
They determined that the creatures use a number of "unconventional aerodynamic
mechanisms to generate force." What is more astounding, writes Rafal Zbikowski
of Cranfield University in an accompanying commentary, is that "the butterflies
appear to switch effortlessly among these mechanisms from stroke to stroke."
Indeed, he concludes, if engineers ever succeed in understanding just how
insects exercise control over such a wide range of abilities, "there will
be a revolution in aeronautics." --Sarah Graham [Scientific American December
12, 2002 ]
Theology and Chaos
| The Self
Organizing Universe |
 |
MP3 130K |
 |
MP3 214K |
|

File Info: Created --/--/-- Updated 25/08/2012
Page Address:
http://leebor2.100webspace.net/Zymic/beffect.html
 The
"Butterfly Effect" is the propensity
of a system to be sensitive to initial conditions.Such systems over
time become unpredictable,this idea gave rise to the notion of a butterfly
flapping it's wings in one area of the world,causing a tornado or some such
weather event to occur in another remote area of the world.
The
"Butterfly Effect" is the propensity
of a system to be sensitive to initial conditions.Such systems over
time become unpredictable,this idea gave rise to the notion of a butterfly
flapping it's wings in one area of the world,causing a tornado or some such
weather event to occur in another remote area of the world.
 discovery of
discovery of
 Super computers run several models of the weather in
parallel to discover whether they stay close together or diverge away from
each other.Models that stay similar in nature give an indication that the
weather is relatively predictable,and are used to indicate the confidence
level that Meteorologists have in a prediction.
Super computers run several models of the weather in
parallel to discover whether they stay close together or diverge away from
each other.Models that stay similar in nature give an indication that the
weather is relatively predictable,and are used to indicate the confidence
level that Meteorologists have in a prediction.
 Animal populations may also be subject to the same
phenomena.Work done by
Animal populations may also be subject to the same
phenomena.Work done by  non-linearity.In certain cases chemical
feedback produces effects as that in
non-linearity.In certain cases chemical
feedback produces effects as that in  "
" Feigenbaum
Feigenbaum